Coaxial Resonators
Cavity filters, or more correctly coaxial resonators, are the cornerstone of good repeater performance. However, there appears to be some sort of black magic in the way they work or how they achieve their performance.
A coaxial resonator is much like a quarter wave stub but open-ended. It can also be likened to a quarter wave antenna enclosed within a conducting chamber and a resonant L/C tuned circuit, encompassing many of the features of each.
In most coaxial resonators the "antenna" is excited by a magnetic coupling loop at the resonant frequency and energy is removed by another loop placed next to the base of the centre resonator. The centre resonator is surrounded by air and there is in fact an optimum ratio of inner case diameter to outer diameter of the resonator of 3.25, which works out to give a characteristic impedance of around 70Ω
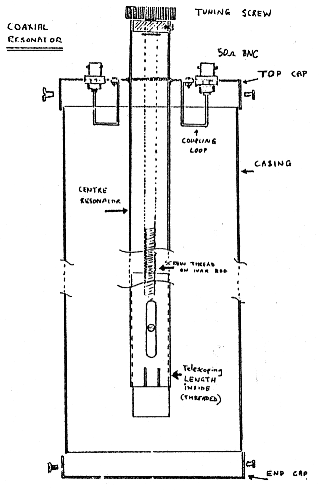
Tek 1 - Construction drawing cavity.
Factors affecting coaxial resonator performance
1. Diameter of centre resonators
This is chosen to achieve an inner resonator to outer casing diameter of 3.25, arriving at the optimum 70Ω impedance. This gives the highest available Q.
2. Length of resonator
This determines the operating frequency of the formed tuned circuit. Length is inversely proportional to frequency, much like any antenna.
3. Coupling loops
These are the means by which energy is input or extracted from the tuned circuit. Loop size determines the amount of coupling. Two major factors have a pronounced effect on coupling: (a) the length of the coupling loop wire adjacentto the centre resonator. The longer this is the more of the energy field around the resonator is intercepted hence a greater influence this has on the coaxial resonator's performance. The longer this wire or loop is next to the centre resonator the higher impedence the input loop becomes; and (b) the spacing of the coupling loop to the centre resonator - ie, the closer the above mentioned loop, the more stronger field it intercepts around the centre resonator, the greater influence it has on tuned circuit performance. The reverse is also true with respect to loop-to-resonator spacing.
It is the manipulation of these two factors that plays a major part in obtaining desired performance of coaxial resonators and generates the biggest headache and level of frustration. For it is with these factors that filter loss, bandwidth and notch depth are juggled.
4. Materials of construction
Most commercial coaxial resonators are constructed of copper but there is an increasing use of aluminium as a casing material. The actual resonator itself, and quite often the inside of the casing, will be silver-plated to increase electrical conductivity, reducing "skin loss" and hence assisting in the maintenance of high Q. The coupling loops are of silver-plated copper and the centre resonator may even be gold-plated at the far end, in the region of high RF impedance.
The centre resonator is usually silver-soldered to the top-cap and the far end of the casing closed off with a copper endcap. The top-cap and end-cap are usually secured to the casing by machine screws or even soldered once all adjustments have been made.
5. Frequency of operation
This is governed by the electrical length of the centre resonator and is usually very close to a quarter wavelength at the frequency of interest. This central portion has within it a screw mechanism by which a telescoping metal tube at the free end of the coaxial resonator is able to effect its mechanical and electrical length, and hence its operating frequency. Most high electrically conductive metals have large coefficients of thermal expansion hence it is possible for the length of the centre resonator to change with extremes of temperature and more importantly change its resonant frequency. To combat this effect commercial designs incorporate a threaded rod of invar, an alloy with an extremely low expansion coefficient which holds the length change to a minimum over extremes of temperature.
Modifications and performance
The set of coaxial resonators used in this article were ex-government (now ofcourse corporatized) made by TCA of Australia, 100 MHz versions type 1564/101/1NZ.
They had been cut down in length externally and internally to bring the operating frequency up to 146 MHz band. The outer casing had a finished length of 610 mm, the centre resonator length ended at 510 mm at 144.350 MHz, but allowed some adjustment length for other frequencies. The resonator itself and its screw thread mechanism were somewhat difficult mechanically to modify. The resonator was removed from the top-cap to achieve this.
The original coupling loops of 100 MHz coaxial resonator are designed for 50 ft in and out, hence to use them for 146 MHz scale their dimensions by 100/146 in overall length parallel to the resonator. The coupling loops were re-connected to the BNC sockets and top-cap. The spacing between the pick-up loop and centre resonator controls the degree of coupling energy into and out of the tuned circuit at its resonant frequency.
Experimental section
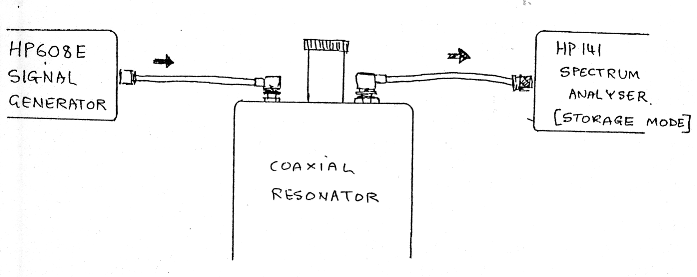
Tek 2 - Set up for bandpass/inductive short/capacitive short/notch.
With the aid of a spectrum analyser in storage mode and a wide range signal generator, with a constant output over the swept frequency, the characteristics of coaxial resonators on the 2 m band can be examined. The frequencies of interest were the linear repeater input and output frequencies for which these resonators were modified. In the test set up the 50 Ω generator feeds the coaxial resonator input and output feeding the spectrum analyser.
1. Bandpass performance
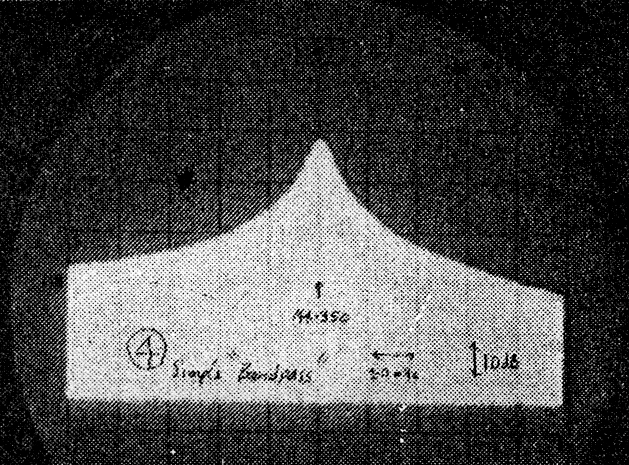
Fig 1
Sweeping the signal over 10 MHz produces the response of Figure 1. The 3 dB points are about 200 kHz and insertion loss under 0.5 dB. When you look at the response of this tuned circuit you see the attenuation is very poor 600 kHz away from centre frequency. Clearly this set up would be very inadequate for repeater filtering purposes to keep the transmitter out of the receiver.
2. The anti-resonant notch

Fig 2
There is a simple modification to this bandpass configuration that will produce the required frequency response. A notch at the unwanted frequency of the transmitter to keep it out of the receiver is obtained by placing a small value capacitor (2-20 pF variable trimmer) across the input and output loops (Figure 2). This produces an amazing effect. Figure 3 shows a notch in the swept response below the passband peak. The notch depth depends on the Q of the tuned circuit. Compare this to the simple band-pass response. On first examination the capacitor looks like a short-circuit across the loops, but the overall summation of phase shift across the capacitor and phase shift across the tuned circuit, both frequency dependant, produces this curious but very useful effect. The amount of capacitance controls the peak-to-notch frequency difference; more capacitance brings the notch and peak closer together while less spreads them further apart. How close one can get them depends entirely on the overall Q of the system. Circuit Q depends directly on the amount of coupling hence, in an overcoupled resonator with its inherent very low insertion loss, it will be very difficult to achieve a close peak-to-notch with good rejection notch depth.
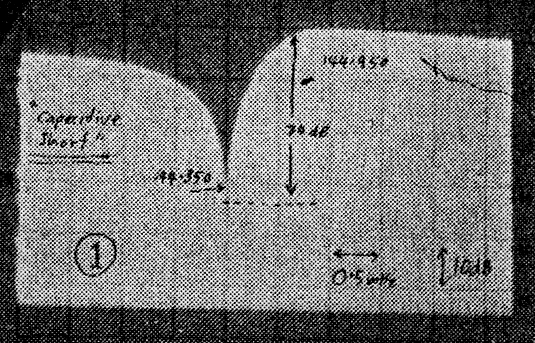
Fig 3

Fig 4
The same phenomena occurs with a "shorting conductor" or a "shorting coax" (Figures 4 and 5). However, the passband peak and rejection notch are reversed in frequency position (Figure 6). The peak to notch separation is once again controlled by the amount of inductance; the more inductance the wider the separation between notch and peak, while less inductance allows them closer together. Once again the depth of the rejection notch and separation are controlled by the circuit Q. This effect can be obtained by using a piece of 50Ω coax between the input and output. Its length governs the peak to notch separation in the same way as does the shorting inductor (Figure 5).
Fig 5
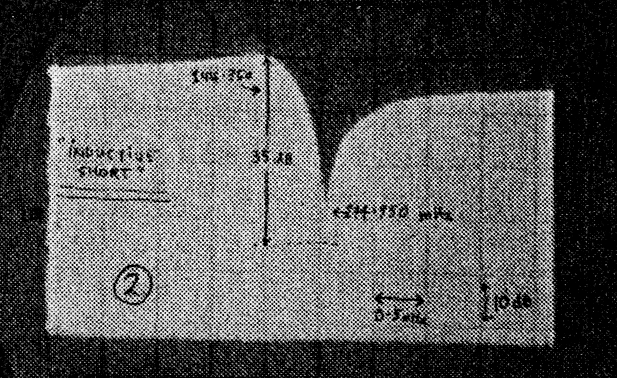
Fig 6
3. Suckout Notch
Tek 3 - Notch configuration.
This is the opposite of the bandpass response and the coaxial resonator is placed in the feedline as is shown. At the frequency of resonance the tuned circuit begins to accept energy from the coax feed reducing the amount of power reaching the spectrum analyser, hence the dip in the swept response. However, as you can see, the overall depth of the notch is only 18 dB. This depth is governed by the amount of coupling; the greater the coupling the deeper and broader the notch becomes, the less the coupling the shallower and the narrower it becomes (Figure 7).
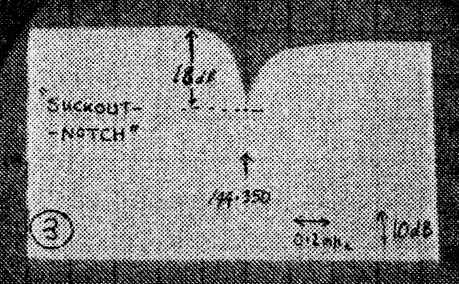
Fig 7
Coaxial resonators designed with the same input and output impedance can be coupled together to perform various bandpass configurations. Placing them in series will add losses and add notch depth if they are on identical frequencies.
By placing several coaxial resonators in series with passbands and rejection notches in the correct places one can get enough isolation between a transmitter and receiver to run them both off the one antenna. This is called duplexing and the group of connected coaxial resonators called a duplexer. Transmitter to receiver isolation figures are measured in decibels and one usually requires at least 80 dB to achieve duplexing.
In a repeater set up (eg, transmitting on 144.350 MHz and a receiver on 144.950 MHz) the connection of coaxial resonators would be as follows: for receive coaxial resonators are tuned to pass 144.950 MHz and have "capacitive shorts" between input and output connectors to place a rejection notch at the transmitter frequency of 144.350 MHz. This keeps the transmitter from overloading the receiver and requires several resonators connected in series to achieve this.
On the transmit side the coaxial resonators are required to pass 144.350 MHz and reject 144.950 MHz. It may seem silly rejecting the repeater input frequency from the transmitter but a transistor or even (dare I say) valve transmitter has a broad noise pedestal centred symetrically around the carrier and, at 600 kHz awayfrom the carrier, the noise level generated by the transmitter may still be in the millivolt level. The rejection notch at the receive frequency attempts to keep noise off the input frequency and let the receiver respond to the wanted microvolt signals that would normally be buried in the transmitter noise.
A novel feature of coaxial resonators is the phenomenon of harmonic resonance. A resonator tuned for 144.350 MHz functions well at the 3rd harmonic at 433.050 MHz as it is a three-quarter wavelength structure. However, it is over-coupled at 70 cm using 2 m coupling loops. At 288.7 MHz, or 2nd harmonic, the resonator exhibits a shallow notch a few decibels deep due to the resonant half wave structure.
The same occurs for the inductive and capacitive "loaded" coaxial resonators. The message is that although the circuits are very high Q they will not eliminate harmonics and you still need a low pass filter on the transmitter. Running these three-quarter wave resonators at 70 cm yields another problem - thermal drift. Because the resonators are three times as long thermal expansion will be three times as great, hence they are going todrift much more in frequency with temperature changes.
Well, that's a very simplistic look at the coaxial resonator and its usefulness. There are other variations of it, in the form of loaded versions and helical versions, but they all are very closely related in the resonant family. For greater understanding of them you should have a look at the references, especially the FM and Repeaters by ARRL. If I can be of any assistance to anyone contemplating building a set of coaxial resonators I would be more than happy to help in any way I can. The first time round they can be a great source of frustration and loss of hair by manual means.
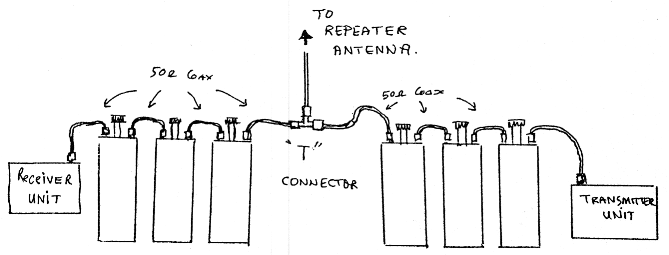
Tek 4 - Repeater duplexer configuration.
References
- RSGB, VHF UHF Manual, 4th Edition
- "A Lo Cost PC Board Duplexer", QST, April 1979.
- ARRL, FM and Repeater Handbook
- K. Wiener DJ9HO, The UHF Compendium, Parts 1 and 2.
- Bitter Experience
The photographs don't show the full depth of the notches in the responses due to the wide sweep width and phosphor blur on the screen. On narrow sweeps the depths of the notches are apparent but the overall shape of the tuned circuit response is not seen.
ZL1BTB, Mike Penfold